Thanks to GPS data of gravity intensity as well as of geopotential (14000 for the State of Baden-Württemberg) are now available with high precision position information. Such a position oriented data base asks for a new concept of Gauß-Listing Geoid computation which is presented here. Since GPS position information is based either on Gauß "surface normal" ellipsoidal coordinates or on Lamé-Jacobi "spheroidal" ellipsoidal coordinates w.r.t. a properly chosen World Geodetic Datum / WGS84 - ITRF96 - WGD2000 / International Reference Ellipsoid / we have chosen a "spheroidal" ellipsoidal expansion of the reference gravitational potential field of degree / order 360/360 (130, 321 coefficients) in terms of associated Legendre functions of the second kind.

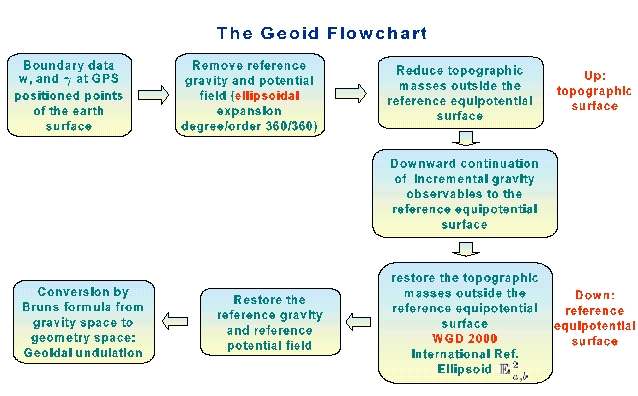
In the first remove step such a spheroidal gravity field expansion including the centrifugal term is applied to reduce both types of data sets / gravity potential - gravity field intensity. The second remove step is built on the terrain effect, namely the removal of the gravity field (potential / field intensity) of topographic masses on top of the Gauß - Listing Geoid / approximated by the International Reference Ellipsoid /. Such a reduction of topographic masses aims at a genesis of a harmonic incremental potential / disturbing potential / outside the Gauß - Listing Geoid, namely the International Reference Ellipsoid. The reduced surface data are downward continued from the Earth topographic surface by means of the regularized inverse Dirichlet Boundary Value Problem: Given the incremental potential on the International Reference Ellipsoid the forward external, ellipsoidal Dirichlet Boundary Value Problem is solved by means of an ellipsoidal Green function, also called ellipsoidal Abel - Poisson kernel. The ellipsoidal Abel - Poisson integral maps the incremental potential on the International Reference Ellipsoid to an incremental potential in the external space, for instance to points on the Earth surface. The inverse Abel - Poisson integral of ellipsoidal type as well as its gradient norm is used to convert incremental potential data as well as incremental gravity data from the surface of the Earth to incremental potential data on the International Reference Ellipsoid. Such an improperly posed problem is solved by (i) discretization of both the integral equations of the first kind and by (ii) Tykhonov-Phillipps- Regularization (Best Linear Uniformly Minimum Bias Estimation: BLUMBE). The regu larization factor is chosen by the minimum of the Mean Prediction Error MSPE (E.Grafarend and B.Schaffrin: Ausgleichungsrechnung in linearen Modellen, BI Wissenschaftsverlag, pages 123-126, § 2 (c), Mannheim 1993).

As soon as the downward continuation operation of the boundary data of type incremental potential and incremental gravity is concluded, the restore procedures start. In the first restore step the terrain effect on the International Reference Ellipsoid is recomputed. The second restore step is built on a computation of the spheroidal gravity potential field to degree / order 360/360 (130, 321 coefficients, see the Internet http://www.uni-stuttgart.de/gi/research/index.html#Projects including the centrifugal potential on the International Reference Ellipsoid. Finally all constituents of the incremental potential on the International Reference Ellipsoid are converted via the ellipsoidal Bruns formula into geoidal undulations. See the attached flow chart for the Geoid computation. From potential data on the Earth surface a potential Geoid is computed while from data of gravity field intensity a gravimetric Geoid is produced. These two solutions for Geoid computation leave us with the problem to compute a "weighted Geoid" from both data sets. The problem of proper weighting is addressed. Case studies from data sets representing the State of Baden- Wuerttemberg / the potential Geoid - the gravimetric Geoid / highlight the new ellipsoidal Geoid computation.

Actually I would like to dedicate my contribution to the memory of Kresimir Colic with whom I cooperated at his time at Bonn University in the late sixties / early seventies of last century.